R
RAYTHEON23
Гостин
pa grcite velat komunjarite ne izmislija...da beee. oni se izmislija:pos2::pos2:Vremeto go izmislija komunjarite...
pa grcite velat komunjarite ne izmislija...da beee. oni se izmislija:pos2::pos2:Vremeto go izmislija komunjarite...
од оваа теорија и тие пред за времето како посебна димензија испаѓа дека иднината е предодередена од минатото и она што се случува во сегашноста..дали мислите дека иднината е фиксно детерминирана од минатото и сегашноста???Е сега после тоа што го кажа Тhe Goddess, ај да кажеме сегашноста потекнува од минатото и не води во иднината која, каква ќе биде зависи од сегашноста (со повеќе точки се добива права, со повеќе прави добиваме рамнина, и на крај тридимензија). а твоето со кружницата знаш како на најприост начин го сватив по се она прочитано, ако ја ротираме околу нејзин пречник ми асоцира на природата, енергијата во неа кружи, таа никогаш не умира, само преминува од еден во друг облик.
Дали мислиме дека постои „судбината“?од оваа теорија и тие пред за времето како посебна димензија испаѓа дека иднината е предодередена од минатото и она што се случува во сегашноста..дали мислите дека иднината е фиксно детерминирана од минатото и сегашноста???
Ова е премногу за мојот скромен коефициент на интелигенција...ако сакам да ги сфатам, и ако воопшто можам да ги сфатам, ќе треба подолго да ги гледам и да размислувам за нив...За да му биде појасно на Агора, забелешка-четвртата и петтата слика се дводимензионални проекции на четири и пет димензионално тело.
GUT agora znaci Gospod na germanski a ako go prevedeme na angliski e great unified theory znaci nema zakon vo fizikata koj gi obedinuva 4-te osnovni sili(elektro-magnetna.gravitacija,jakata i slabata nuklearna energija)znaes imalo eden filozof matematicar Laplas toj go oformil pravecot narecen DETERMINIZAM spored koj moze odnapred se da se presmeta TOTALNO se...arno ama koga po makotrpna rabota ne uspeal se razocaral..inaku seuste ja baraat taa ravenka-naverojatno treba da se inkorporira temnata materija i energija ama i da uspeeme vo toa sigurno ke fali nestoДали мислиме дека постои „судбината“?
Се разбира дека постои.
Ако неможеме да ги менуваме законите на физиката, неможеме да ја менуваме ни иднината, затоа што сите честички и се` останато во овој универзум, и сите процеси, ќе се одвиваат по законите на физиката...
Ме мрзи да го дообјаснувам ова...
Јасен бев?
ако не, тогаш попосле повеќе ќе објаснам...


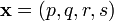




Знаеме дека материјата е изградена од енергија, веројатно во облик на електромагнетни бранови.
Ќе се движат електромагнетните бранови кои ја градат материјата.
топлината е еден од многуте видови на енергија, а температурата мерна единица за топлина
Електронот ( и протонот, неутронот,т.е. секоја честичка ) е „изграден“ од електромагнетни бранови.
температурата ни покажува колку топлина има во нешто
Но, ајнштајн веројатно брзината на светлината ја сфача бреку магнетната пропустливост и диелектричната константа на вакуум, со онаа формулата: ц=1 врз квадратен корен од м.п. пати д.к. на вакуумот. ( мислам, ама не сум сигурен дека пред коренот го имаше пи )
Нели јас докажав дека е паралелна димензија, или не?
Почнав димензиите ( должина, ширина, висина и т н ), преку точка, права, рамнина, простор и т н, да ги гледам како еквивалент на времето.
Како што точката нема должина, така и сегашноста нема траење...
и.т.н. Нели тоа е доказ?
Првичната дефиниција за паралелна димензија нека биде:
Паралелна димензија е она што има исти/слични карактеристики со класичните димензии...
На ТОЧКАТА, која нема ниту една класична димензија, еквивалент, паралелна димезија, можеме да ја земеме СЕГАШНОСТА ( или секој оној дел од времето кој нема траење ).
На она што има една класична димензија, правата, како еквивалент, како паралелна димензија можеме да ги земеме ВРЕМЕТО ШТО ИМА ТРАЕЊЕ ( човекот не знаепосебен збор за ова...или знае? ).
За еквивалент на она со две и три димензии, рамнината и просторот, паралелна димензија за нив, човекот уште не знае, треба да го смислиме...ајде сите заедно да го смислиме, па ќе ја поделиме нобеловата награда, важи?
А ако се промени м.п. и д.к. на вакуумот, ќе се промени и брзината на течење на времето
Една секунда се дефинира како времето за кое светлината ќе помине 300 000 километри
значи, имаме две познати временски димензии, а тоа се: сегашноста, еквивалентна на точка, времето како што го знаеме, еквивалентно на права
Ok, vaka, najdobronamerno imam edno prasanje do tebe, zosto stvarno me zainteresira. KOLKU GODINI IMAS?Дали мислиме дека постои „судбината“?
Се разбира дека постои
kazimi sto mislis, i ke ti kazam?Ok, vaka, najdobronamerno imam edno prasanje do tebe, zosto stvarno me zainteresira. KOLKU GODINI IMAS?
Си направил добра колекција на постови на годинатаOk, vaka, najdobronamerno imam edno prasanje do tebe, zosto stvarno me zainteresira. KOLKU GODINI IMAS?

Од тоа може само да се види дека неможам накратко да објаснам некои работи...но не и дека немам познавања од областа на физиката...Си направил добра колекција на постови на годината
Iskreno da ti kazam, koga te citav tebe i tvoite postoi mi se vrati filmot nekolku godini unazad, i se setiv na moite tinejdzerski denovi, 14-15 godisen, koga gi citav cickovcite Ajnstajn i Hoking, koga mislev deka zad poimot "postoenje" se krijat astrofizika, kosmologija, kvantna i nuklearna fizika, koga cela vecer ne spiev i se obiduvavav da analiziram se i sesto, i sekoja moja napredna faza vo moite mislenja ja smetav za genijalna, a pri toa zaboravav deka blage veze nemam od matematika a najmnogu sto znam e da resam kvadratni ravenki i cat-pat nekoi poslozeni sistemi i integrali.kazimi sto mislis, i ke ti kazam?
Најомилената реченица на NEBO666 како изговор за неговата шизофренија.п.с. Ајнштајн додека бил студент имал најниски оценки...
Најомилената реченица на NEBO666 како изговор за неговата шизофренија.



Целта не ми беше да докажам нешто.Барем главната цел не би беше тоа.Целта ми беше да ни биде забавно/интересно на сите.Iskreno da ti kazam, koga te citav tebe i tvoite postoi mi se vrati filmot nekolku godini unazad, i se setiv na moite tinejdzerski denovi, 14-15 godisen, koga gi citav cickovcite Ajnstajn i Hoking, koga mislev deka zad poimot "postoenje" se krijat astrofizika, kosmologija, kvantna i nuklearna fizika, koga cela vecer ne spiev i se obiduvavav da analiziram se i sesto, i sekoja moja napredna faza vo moite mislenja ja smetav za genijalna, a pri toa zaboravav deka blage veze nemam od matematika a najmnogu sto znam e da resam kvadratni ravenki i cat-pat nekoi poslozeni sistemi i integrali.
Brat, lugeto cel zivot studiraat i istrazuvaat, trosat milijardi dolari, i povtorno ne se sigurni dali dokazale nesto, a ti so nekolku recenici onaka katapultirani od tvojot mozok sakas da ne uveris nas vo nesto, i pri toa toa da go smetas kako dokaz, a istovremeni i da kazuvas smesni bezmislici so koi samo go pokazuvas tvoeto osnovno nepoznavanje na nekoi osnovni stvari vo naukata. Zatoa zemi procitaj sto e dimenzija, sto e edinicina, sto e fizicka velicina, sto e fizicko pole, sto e materija, sto se nukleoni, sto znaci mojot nickname, sto e spin na cestica, sto e funkcija, sto e laplasoviot determinizam i kako hajzenbergovit princip go otfrla nego, resavaj izvodi i integrali, i posle pak pocni da mislis za ovie stvari , no nikogas , ama bas nikogas nemoj da reces deka si DOKAZAL nesto. Smesno e.
Ne ja osporuvam tvojata intelegincija, sepak, realno kazano interesno razmisluvas, sto e najvazno ramisluvas, i mozebi vo nekoi stvari se soglasuvam so tebe, ama ipak vo naukata si postojat onaka dobro definirani i oddeleni etapi, koi sto nuzno e da gi pomines, a promasis edna, ti otide jaban celiot trud.
